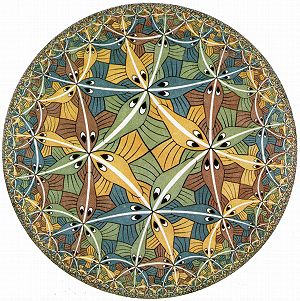
For centuries, people believed that Euclidean geometry was the only conceivable geometry. Then in the early 1800s, Bolyai and Lobachevsky daringly proposed an alternative: hyperbolic geometry. In this course, we study the three most important 2-dimensional geometries: euclidean, spherical, and hyperbolic. Our methods will be those of calculus and our goal will be to show that every surface supports one of these three important geometries. As time allows we'll start investigating topics of current research and applications of non-euclidean geometries.
- Exam 1. Due Monday, March 10 at 5 PM.
- Exam 2. Due Thursday, April 17 at 5 PM. Please be sure to read and sign the rules. Updated!