MA177, Fall 97---Speed and Distance

The point of this worksheet is to get you to think about the relation between speed and distance in various ways, and thus (I hope) to motivate the notion of a derivative. We start out with easier problems, and make them harder as we go along.

1. Suppose Jessica rides her bike at constant speed, and covers 5km in 25 minutes.

1. What is her speed?

2. If we draw a graphical representation of Jessica's trip by plotting her position at each time, how can we ``see'' her speed in the graph?

3. What happens if we drop the assumption that her speed is constant? Does the question still have an answer? Can you interpret what happens from the graphical point of view?

2. Let's reverse the problem: suppose we know that Jarrod rides his bike at a constant speed of 17km/h for 35 minutes.

1. How far does he go?

2. If we want to draw a graphical representation of Jarrod's trip, we have to do it by plotting the information we have, his speed at each time. What will the graph look like? Can you ``see'' how far he goes in the graph?

3. Consider now a different situation: Bryn throws a grapefruit straight up. It goes up for a while, then starts to come down and splats itself on the ground. Colin makes a movie of the whole action, makes careful measurements of the grapefruit's height h at each time t, and comes up with the following table:

t (sec)	0	1	2	3	4	5	6
h (ft)	6	90	142	162	150	106	30

1. Does it make sense to talk about the speed of the grapefruit at a given time? For example, does it make sense to ask how fast it is moving when t=3?

2. Suppose we do what we did before, and consider divide the total distance between when we started filming (t=0) and when we stopped (t=6) by the time interval:

   

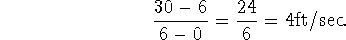
   What is the meaning of this number?

3. What if we do the same computation over a smaller interval, say between t=2 and t=3: what answer do we get, and what does it mean?

4. Can we use a graphical method to do this problem too? What happens when we try? How can we ``see'' the numbers we computed in terms of the graph?

4. Richard and Darcy get tired of this game and consult an oracle. The oracle tells them that after t seconds have elapsed the height of the grapefruit is given by

h = 6 + 100t -16t².

1. Check that this formula does give the numbers we had measured. Why is it better to have the formula than to have the table?

2. What happens when t=7? What does it mean?

3. Suppose we want to know the speed of the grapefruit when t=3. What should we do?

4. Let's make a graph as before, plotting the height at each time t. What does the graph look like? How does it relate to the one in question 3? In terms of the graph, what does your procedure in part 3 above mean?

5. Can you compute the speed of the grapefruit at any time?

6. Can you find a formula for the speed of the grapefruit at any time?

5. Suppose we have a spherical balloon into which we are slowly pumping air, so that the volume of the balloon is growing at the rate of 10 cubic centimeters per second. When we first start watching it, the radius of the balloon is 2 cm.

1. Do you remember the relation between the radius of the balloon and its volume? What is the initial volume of our balloon?

2. When one second goes by, the volume is 10 cm³ bigger. How much bigger is the radius?

3. Is the radius changing at a constant rate?

4. There clearly is a relation between the rate of change of the volume and the rate of change of the radius. Can you figure out what that relation is?